National Puzzlers' League

National Puzzlers' League -- Form Notation
By Lucifer et.al.

Introduction

This page describes a form notation that is used by some NPL members for discussion of form shapes. It's never used in The Enigma to describe forms, so there is no need for you to learn it if you're not interested. However, it is a convenient short-hand for describing form shapes in a medium such as email, and as such you may occasionally see it used on the NPL's mailing list, npl-folk.

Basic halfsquare notation

The atomic unit used to describe a form is a half-square. There are four possible orientations for a halfsquare, and they each have their own symbol. Here they are:

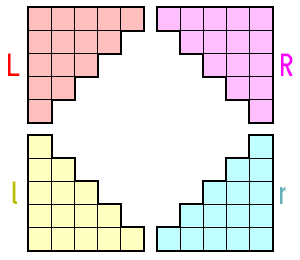L : X X X X X R : X X X X X X X X X X X X X X X X X X X X X X X X X l : X r : X X X X X X X X X X X X X X X X X X X X X X X X X X X X X

In addition, there is a further symbol for a square:

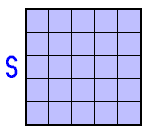S : X X X X X X X X X X X X X X X X X X X X X X X X X

Combining halfsquares

The five symbols above have limited use on their own. They can be combined, however, to define more complex forms. A simple example: rl is a pyramid. You can see this by writing the two symbols out in full:

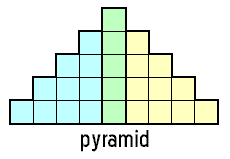X X X X X X X X X X X X X X X X X X X X X X X X X

The column of X's in bold are the overlapping edges of the two halfsquares, "r" and "l". The halfsquares don't have to overlap in this way, as we shall see later, but for the moment we will assume they always do, as that simplifies many forms.

Another example is the rhomboid, which has two forms, rL, and Rl. They are known as the "right rhomboid" and "left rhomboid" respectively. Here's a left rhomboid written out, with the overlapping X's from the R and l in bold, as before:

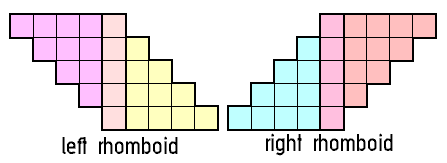X X X X X X X X X X X X X X X X X X X X X X X X X

More complex forms

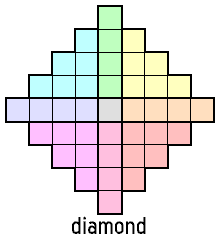
To describe a form such as a diamond, it is no longer possible to just concatenate the symbols already given. The notation for a diamond is "rl:RL", the new symbol is the colon. To understand what the colon means, think about what the notation would mean without it. "rlRL" could be interpreted as follows:

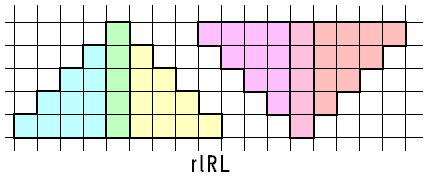X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X

That's to say, it could be seen as just a pyramid (rl) followed by an inverted pyramid (RL). What we want is the following shape (again the overlapping letters are bolded):

X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X

The ":" means "go back and start a new line". To avoid ambiguity, we require that the enclosing grid of squares in which we are placing these symbols must be rectangular. So rl:RL is now unambiguous--two rows, and two symbols on each row, so it must be a 2x2 grid.

The "order" of a form

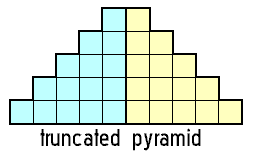
However, this notation will not handle certain forms. For example, a truncated pyramid looks like this:

X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X

This can be represented by rl, if the two halfsquares abut instead of overlap. To indicate this we introduce the idea of the "order" of a form. A form with order 1 has all its halfsquares overlapping by one letter, as in all the examples so far. A form with order 0 has the halfsquares not overlapping at all--so rl, order 0, is a truncated pyramid, and rl, order 1 is a regular pyramid.

Higher orders are possible too, but few standard forms require them.

Windmills and lattices

Three more extensions to the notation are needed to deal with windmills, lattices, and non-standard derivatives of lattices. To describe a windmill, we need a symbol that shows that there are no letters whatsoever in a particular grid square. We use a hyphen, "-", to indicate this, so for example S-:-S is a left windmill:

X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X X

Again I have bolded the overlap, here only one letter.

In a lattice, the "empty" quadrant only has letters along the edge. We can indicate this by using a * after a symbol to show that we only want the symbol to contribute the right angle of letters. A left lattice: SR*:l*S, and here it is:



        X X X X X X X X X

        X X X X X       X

        X X X X X       X

        X X X X X       X

        X X X X X X X X X

        X       X X X X X

        X       X X X X X

        X       X X X X X

        X X X X X X X X X

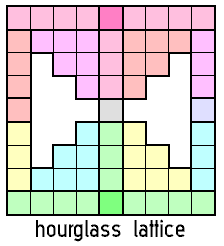
Finally, if you want to include another halfsquare in the same grid square as the right angle of letters indicated by a *, then you need to use a + to indicate that two consecutive symbols occupy the same grid square. For example L*+RL+R*:l*+rl+r* is a non-standard shape one might call an hourglass lattice. Here it is laid out:



        X X X X X X X X X

        X X X X X X X X X

        X   X X X X X   X

        X     X X X     X

        X       X       X

        X     X X X     X

        X   X X X X X   X

        X X X X X X X X X

        X X X X X X X X X

Non-orthogonal layouts

Stars can be represented by --r-:-SSL:rSS-:-L--, but there is a problem. This description produces:



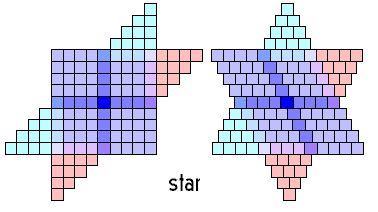                    X    

                  X X    

            X X X X X X X

            X X X X X X  

            X X X X X    

          X X X X X X

        X X X X X X X

            X X          

            X

This is accurate inasmuch as it represents correctly the relationships of the words, but a star is not presented on an orthogonal grid like this. The solution is simply to say in a form's description that it is to be presented on a left (or right) star grid, if appropriate; if no mention is made of the grid then it is assumed to be orthogonal.

Compound forms

To represent compound forms requires another addition to the notation: subscripts. A pair of connected squares, for example, would be written S₁S₂. Laid out:



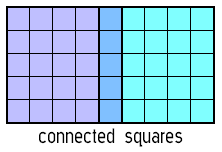         X X X X X X X X X

         X X X X X X X X X

         X X X X X X X X X

         X X X X X X X X X

         X X X X X X X X X

The left hand square is S₁; the right hand one is S₂. With this notation, we can represent much more complex compound forms, such as the Rokeby star: -r₁l₁-:R₂l₂+R₃L₃+r₄L₄:r₅L₅+r₆l₆+R₇l₇:-R₈L₈- This is unambiguous, but an actual Rokeby star will probably help to clarify the notation. Once again, boundary letters are shown in bold, though I have not bolded the letters on the boundaries between two halfsquares in the same grid square. First, a layout showing the overall shape:



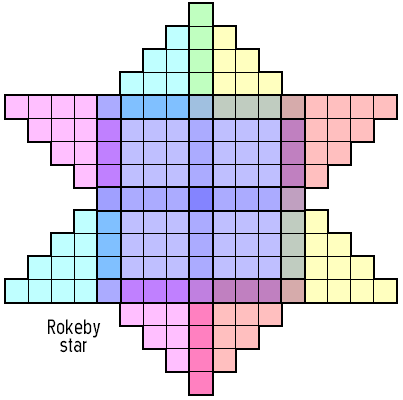                              X

                            X X X

                          X X X X X

                        X X X X X X X

              X X X X X X X X X X X X X X X X X

                X X X X X X X X X X X X X X X

                  X X X X X X X X X X X X X  

                    X X X X X X X X X X X    

                      X X X X X X X X X      

                    X X X X X X X X X X X    

                  X X X X X X X X X X X X X

                X X X X X X X X X X X X X X X  

              X X X X X X X X X X X X X X X X X

                        X X X X X X X    

                          X X X X X      

                            X X X   

                              X

Now, here's a picture showing in bold the boundaries between the constituent forms, rather than the halfsquares referred to by the notation. As you can see, the Rokeby star is made up of two pairs of connected pyramids, one on top of the other, with a pair of rhomboids on each side.



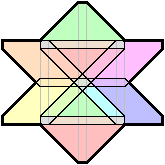                              X

                            X X X

                          X X X X X

                        X X X X X X X

              X X X X X X X X X X X X X X X X X

                X X X X X X X X X X X X X X X

                  X X X X X X X X X X X X X  

                    X X X X X X X X X X X    

                      X X X X X X X X X      

                    X X X X X X X X X X X    

                  X X X X X X X X X X X X X  

                X X X X X X X X X X X X X X X

              X X X X X X X X X X X X X X X X X

                        X X X X X X X

                          X X X X X

                            X X X

                              X

The size of the form

To describe a form shape, the above notation is sufficient. If you actually want to describe a specific form, you have to say what size it is, however. For example, a square of side 7 and a square of side 5 are both just S in the notation as it stands.

The side of the square that forms the underlying grid for the notation is given when you need to define the size of the form--you simply say "N=5" (or whatever it is). For simple forms like squares and halfsquares this is just the same as the side of the form; for more complex forms it is not. For example, a 7-star has N=3; a 10-star has N=4.

A list of form shapes

Here, for reference, is a list of all the standard form shapes described in the form notation. Where a form can exist in rotated and reflected forms I am only giving one version here.

Cambridge hexagon: rS:SL
Connected squares: S₁S₂
Diamond: rl:RL
Enneagon: rl-:RSS:-S-
Halfsquare: R
Hexagon: rSl:RSL
Lattice: SR*:l*S
Octagon: rSl:SSS:RSL
Pentagon: rl:RS
Pyramid: rl
Pyramidal windmill: rl--:--RL
Rectangle: rl-:RSl:-RL
Rhomboid: Rl
Square: S
Star: --r-:-SSL:rSS-:-L--
Windmill: S-:-S

Note that the Pygmy hourglass and the truncated pyramidal windmill cannot be described with the notation. Various more or less esoteric extensions to the notation have been proposed to handle this, but none has been agreed on yet by the people who use the notation.